How To Draw A Hyperbolic Paraboloid
How To Draw A Hyperbolic Paraboloid - Use three of them to form an equilateral triangle. Web 4.7k views 7 years ago drawing quadrics. Most folks find the hyperbolic paraboloid more difficult than the elliptic paraboloid to draw. Take six of the skewers. Web how to make a hyperbolic paraboloid using skewers step 1 cut the skewers to the desired length. Web a couple of ways to parameterize it and write an equation are as follows: Web elliptic paraboloid indicating we have found local extrema. Web sketching quadric surfaces by hand. Hyperbolic paraboloid indicating that we are at a saddle point. Z =x2 −y2 z = x 2 − y 2. Web learn how to draw an elliptic and a hyperbolic paraboloid. Finally, add in the base: A hyperboloid can be generated intuitively by taking a cylinder and twisting one end. And you have a hyperbolic paraboloid! Web about press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features nfl sunday ticket press. We will now restate this test in the context of identifying local extrema. Twist gently and you’ll get a shape somewhere between a cone and a cylinder: Web drawing a hyperbolic paraboloid. Fortunately, as we have seen, there is a second derivative test that does exactly this for us. Step 2 make a regular tetrahedron. Hyperbolic paraboloid indicating that we are at a saddle point. Z = ax2 + by2 z = a x 2 + b y 2 (where a and b have different signs) with just the flip of a sign, say x2 +y2 to x2 −y2, x 2 + y 2 to x 2 − y 2, we can change from an. Web learn how to draw an elliptic and a hyperbolic paraboloid. Web 1 2 3 4 5 6 7 8 9 share 220 views 2 years ago this video shows the elements of the hyperbolic paraboloid and how the plane director/directrix has an. Web a hyperboloid can be made by twisting either end of a cylinder. Web elliptic paraboloid indicating. Z =x2 −y2 z = x 2 − y 2. Web a hyperboloid can be made by twisting either end of a cylinder. Web learn how to draw an elliptic and a hyperbolic paraboloid. Hyperbolic paraboloid indicating that we are at a saddle point. Time to teach you the tricks of the trade. Hyperbolic paraboloid indicating that we are at a saddle point. Identify the parabolas (1/3) a hyperbolic paraboloid is, roughly speaking, a surface that is made up of hyperbolas whose vertices lie on one of two parabolas. Z = ax2 + by2 z = a x 2 + b y 2 (where a and b have different signs) with just the. Step 2 make a regular tetrahedron. Take six of the skewers. Web a couple of ways to parameterize it and write an equation are as follows: Fortunately, as we have seen, there is a second derivative test that does exactly this for us. I've found that skewers often are not quite evenly cut and are rarely the. Note here, as the absolute value of x x increases, the z z. And you have a hyperbolic paraboloid! Most folks find the hyperbolic paraboloid more difficult than the elliptic paraboloid to draw. Web sketching quadric surfaces by hand. Whatever you use, it needs to be durable enough to withstand being folded and unfolded a number of times. Fortunately, as we have seen, there is a second derivative test that does exactly this for us. Use three of them to form an equilateral triangle. Web learn how to draw an elliptic and a hyperbolic paraboloid. Web sketching quadric surfaces by hand. Z = ax2 + by2 z = a x 2 + b y 2 (where a and. Does anyone know what the record is for the most number of times hyperbolic paraboloid has been said out loud in a youtube video? Finally, add in the base: Web how to make a hyperbolic paraboloid using skewers step 1 cut the skewers to the desired length. Web a hyperboloid can be made by twisting either end of a cylinder.. Web a hyperboloid can be made by twisting either end of a cylinder. Use three of them to form an equilateral triangle. Web 4.7k views 7 years ago drawing quadrics. Fortunately, as we have seen, there is a second derivative test that does exactly this for us. We will now restate this test in the context of identifying local extrema. Slices parallel to the x axis and y axis. Fold and unfold the paper in half We see that there are two real solutions for when or equivalently when so, we see that when the pure partials have the same sign, the quadric surface is a hyperbolic paraboloid when and an elliptic paraboloid when when the anaylsis above is insufficient to make any conclusions. Web how to make a hyperbolic paraboloid using skewers step 1 cut the skewers to the desired length. Does anyone know what the record is for the most number of times hyperbolic paraboloid has been said out loud in a youtube video? Material first things first, you need a sheet of origami paper. Note here, as the absolute value of x x increases, the z z. Identify the parabolas (1/3) a hyperbolic paraboloid is, roughly speaking, a surface that is made up of hyperbolas whose vertices lie on one of two parabolas. I'm using a small sheet of gold foil paper, but any origami paper will do. At this point, you should get to know elliptic paraboloids and hyperbolic paraboloids. Web draw the hyperbolas one hyperbola for each of the parabolas drawn in planes perpendicular to the axis upper hyperbola drawn with upper parabola the plane is the upper bound for the u variable for this is the plane x = 4 vertices are on the upper parabola lower hyperbola drawn with lower parabola the plane is the lower bound for.
Hyperbolic paraboloid GeoGebra Dynamic Worksheet

Howto DRAW HYPERBOLIC PARABOLOID (part 3) YouTube
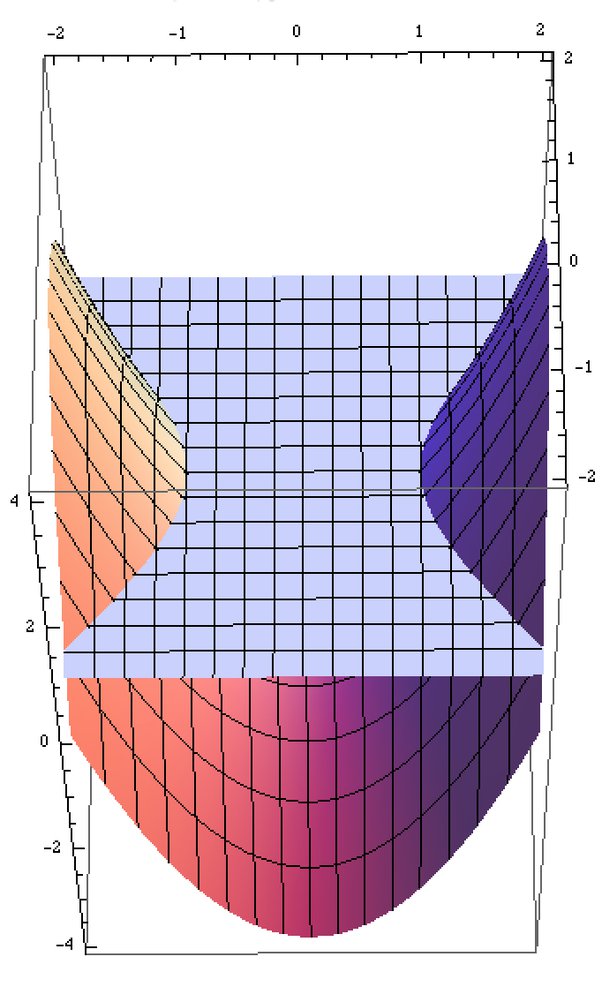
Detail How To Draw Hyperbolic Paraboloid Koleksi Nomer 6

Saddle surface of a hyperbolic paraboloid / Models // Mathematical Etudes

Howto DRAW HYPERBOLIC PARABOLOID (part 2) YouTube
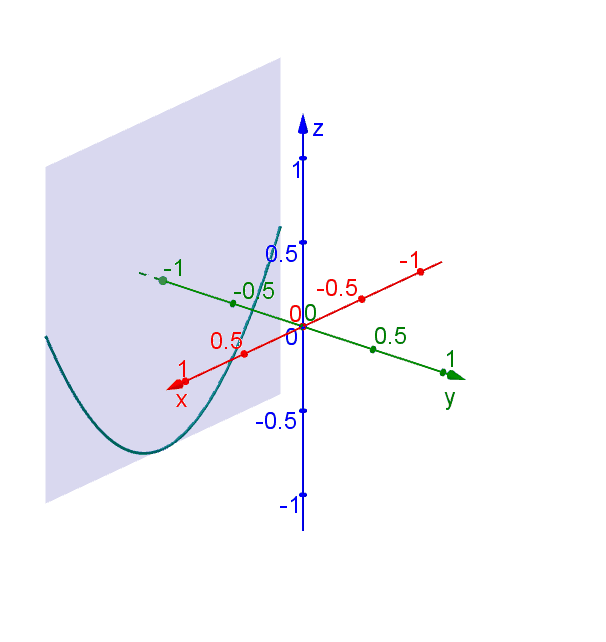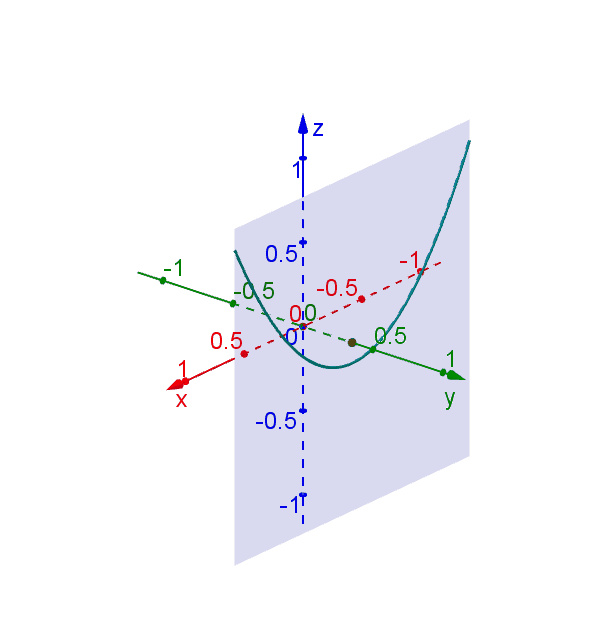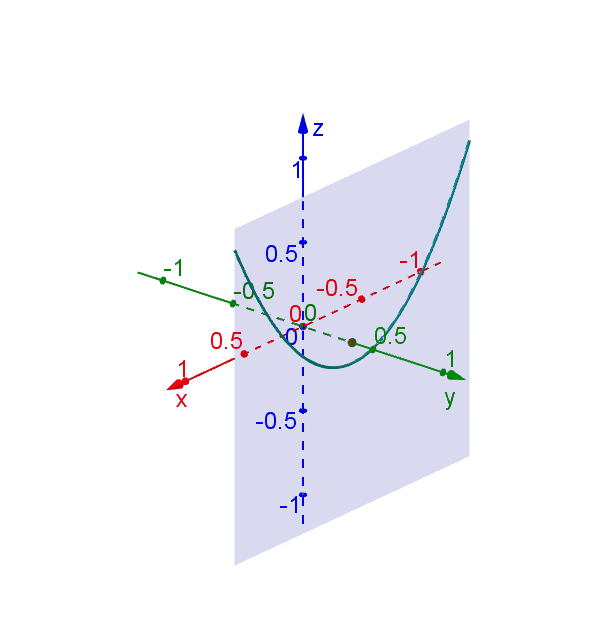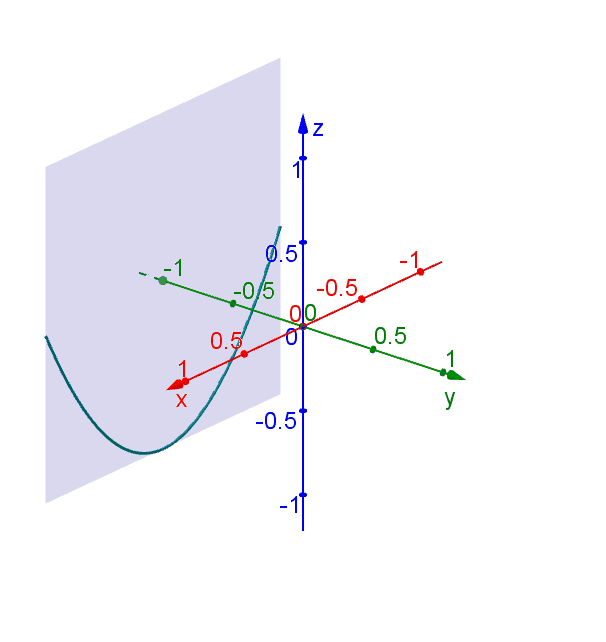
Hyperbolic paraboloid GeoGebra Dynamic Worksheet
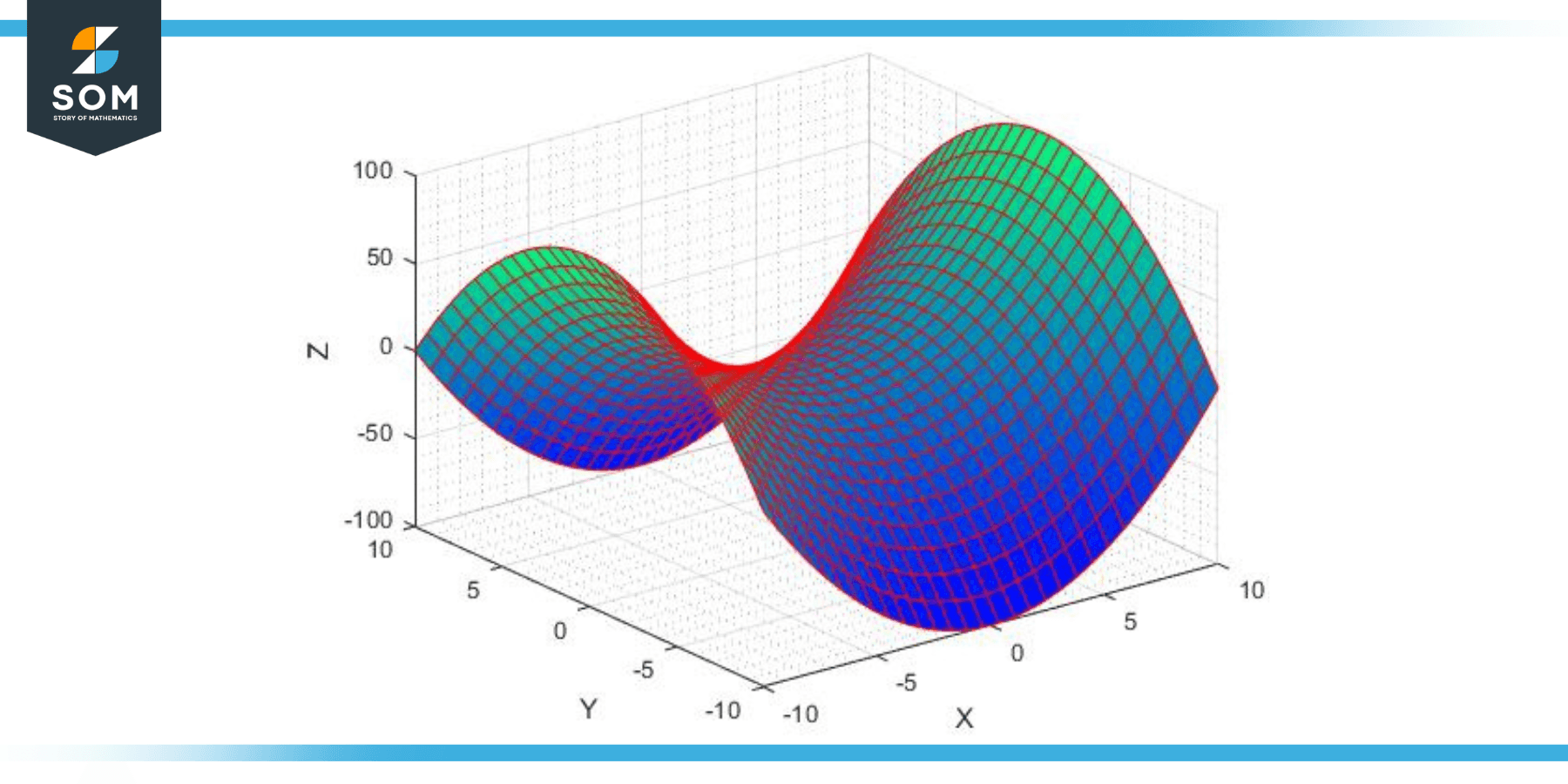
The Hyperbolic ParaboloidDefinition, Geometry With Examples

Howto DRAW HYPERBOLIC PARABOLOID (part 4) YouTube

Quadric Surface The Hyperbolic Paraboloid YouTube

Drawing Hyperbolic Paraboloids YouTube
Whatever You Use, It Needs To Be Durable Enough To Withstand Being Folded And Unfolded A Number Of Times.
Step 2 Make A Regular Tetrahedron.
Twist Gently And You’ll Get A Shape Somewhere Between A Cone And A Cylinder:
Web You Begin By Drawing A Set Of Axis:
Related Post: