How To Draw Slope Fields
How To Draw Slope Fields - Forming a slope field slope fields & equations slope fields & equations google classroom So each individual point of a slope field (or vector field) tells us the slope of a function. The slope, y ' ( x ), of the solutions y ( x ), is determined once we know the values for x. Web share save 3.7k views 4 years ago differential equations learn how to create slope fields and sketch the particular solution to a differential equation. Sketching slope fields slope fields introduction worked example: Match a slope field to a solution of a differential equation. Web let's draw small pieces of some of these tangent lines of the function : Web explore math with our beautiful, free online graphing calculator. Web differential equations > sketching slope fields worked example: Match a slope field to a differential equation. See how we match an equation to its slope field by considering the various slopes in the diagram. If you drop a leaf onto this map, where will it go? Web continuing in this manner, you can draw in a slope field at all the integral grid points indicated to obtain a slope field like the one shown. What does. Web let's draw small pieces of some of these tangent lines of the function : Web to draw the slope field, we sketch a short segment at each point with the appropriate slope. Match a slope field to a differential equation. Slope field from equation google classroom about transcript given a differential equation in x and y, we can draw. Slope field from equation worked example: Discover any solutions of the form y. Web sketching slope fields worked example: That's the slope field of the equation. Web explore math with our beautiful, free online graphing calculator. Web you are essentially correct. So each individual point of a slope field (or vector field) tells us the slope of a function. The pattern produced by the slope field aids in visualizing the shape of the curve of the solution. Web to draw the slope field, we sketch a short segment at each point with the appropriate slope. The. The slope field is a cartesian grid where you draw lines in various directions to represent the slopes of the tangents to the solution. Web explore math with our beautiful, free online graphing calculator. Discover any solutions of the form y. Click and drag the points a, b, c and d to see how the solution changes across the field.. This of course depends on where you drop it. Web share save 3.7k views 4 years ago differential equations learn how to create slope fields and sketch the particular solution to a differential equation. Therefore by drawing a curve through consecutive slope lines, you can find a solution to the differential equation. We give a brief example of sketching a. Plotting slopes at various points, and using isoclines. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Sketching slope fields slope fields introduction worked example: Check the solution boxes to draw curves representing numerical solutions to the differential equation. The completed graph looks like the following: Draw conclusions about the solution curves by looking at the slope field. The slope field is utilized when you want to see the tendencies of solutions to a de, given that the solutions pass through a certain localized area or set of points. Web a slope field is a visual representation of a differential equation in two dimensions. Click and. Slope field from equation google classroom about transcript given a differential equation in x and y, we can draw a segment with dy/dx as slope at any point (x,y). Click and drag the points a, b, c and d to see how the solution changes across the field. That's the slope field of the equation. The most basic way to. Slope field from equation google classroom about transcript given a differential equation in x and y, we can draw a segment with dy/dx as slope at any point (x,y). So each individual point of a slope field (or vector field) tells us the slope of a function. Click and drag the points a, b, c and d to see how. The beauty of slope field diagrams is that. Forming a slope field google classroom about transcript given a differential equation in x and y, we can draw a segment with dy/dx as slope at any point (x,y). And this is the slope a solution \(y(x)\) would have at \(x\) if its value was \(y\). This shows us the rate of change at every point and we can also determine the curve that is formed at every single point. At a point \((x,y)\), we plot a short line with the slope \(f. Discover any solutions of the form y. The slope field is a cartesian grid where you draw lines in various directions to represent the slopes of the tangents to the solution. At each point in a direction field, a line segment appears whose slope is equal to the slope of a solution to the differential equation passing through that point. Slope fields (also called vector fields or direction fields) are a tool to graphically obtain the solutions to a first order differential equation. The slope, y ' ( x ), of the solutions y ( x ), is determined once we know the values for x. Web a slope field is a visual representation of a differential equation in two dimensions. Web you are essentially correct. The slope field is utilized when you want to see the tendencies of solutions to a de, given that the solutions pass through a certain localized area or set of points. Web sketch a slope field for a given differential equation. Web explore math with our beautiful, free online graphing calculator. Web continuing in this manner, you can draw in a slope field at all the integral grid points indicated to obtain a slope field like the one shown.
How to sketch direction fields — Krista King Math Online math help
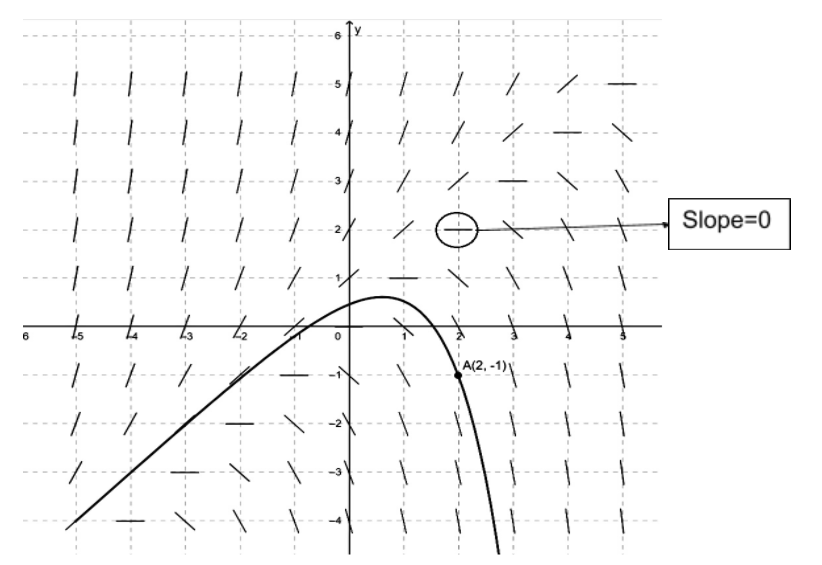
How do you draw the slope field of the differential equation \\[{{y
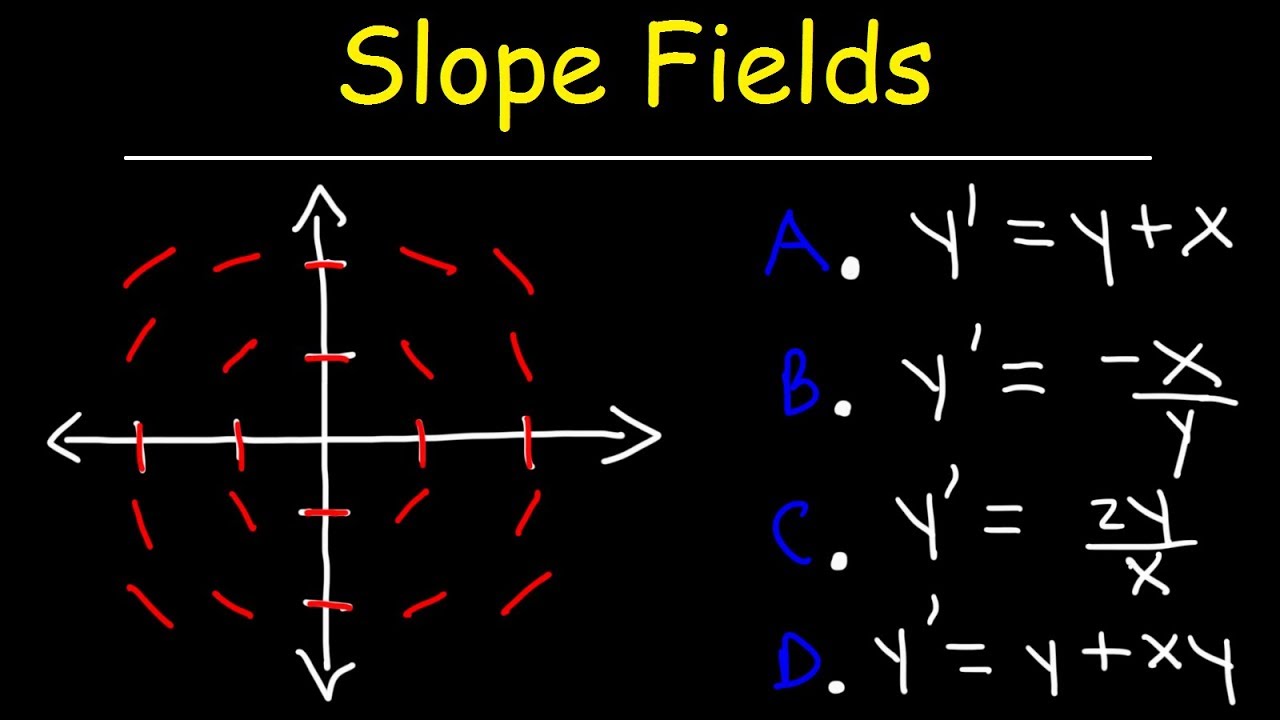
Slope Fields Calculus YouTube

Sketch the slope field and sketch the particular equation YouTube

Slope Fields_Example 2 on how to sketch a slope field YouTube
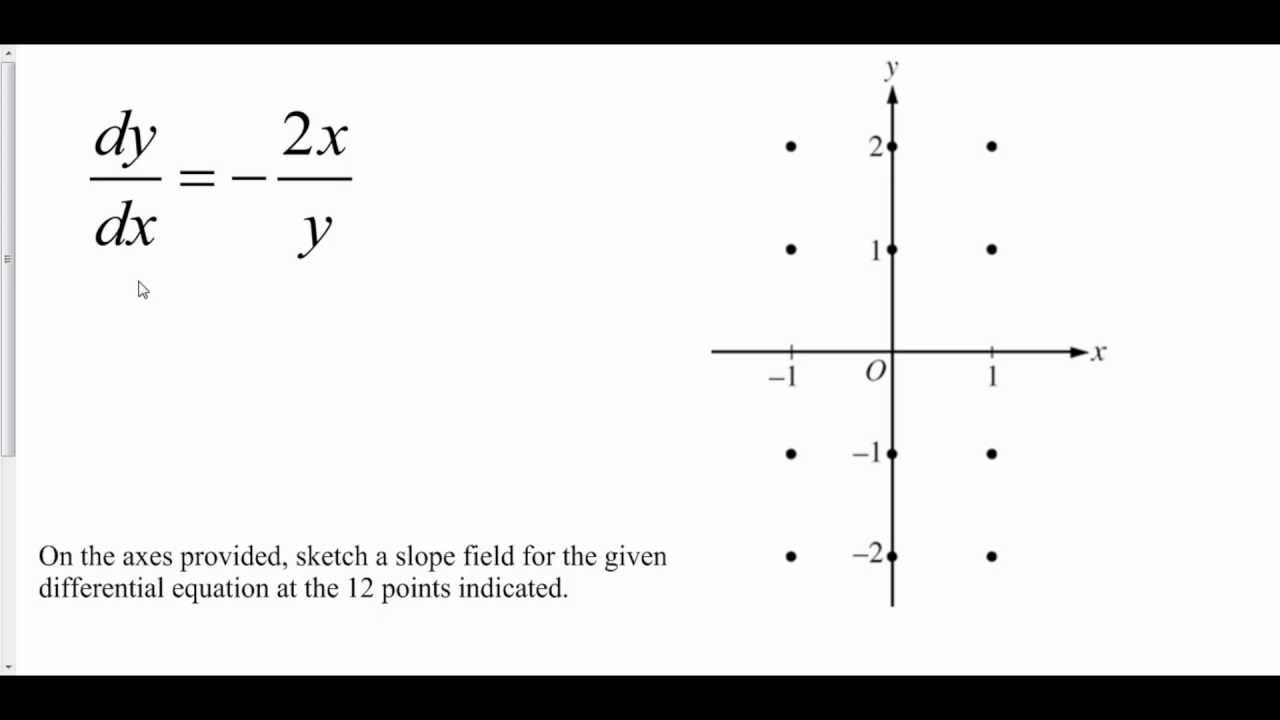
Graphing Slope Fields from a Differential Equation YouTube

Differential Equations How to Draw slope fields YouTube
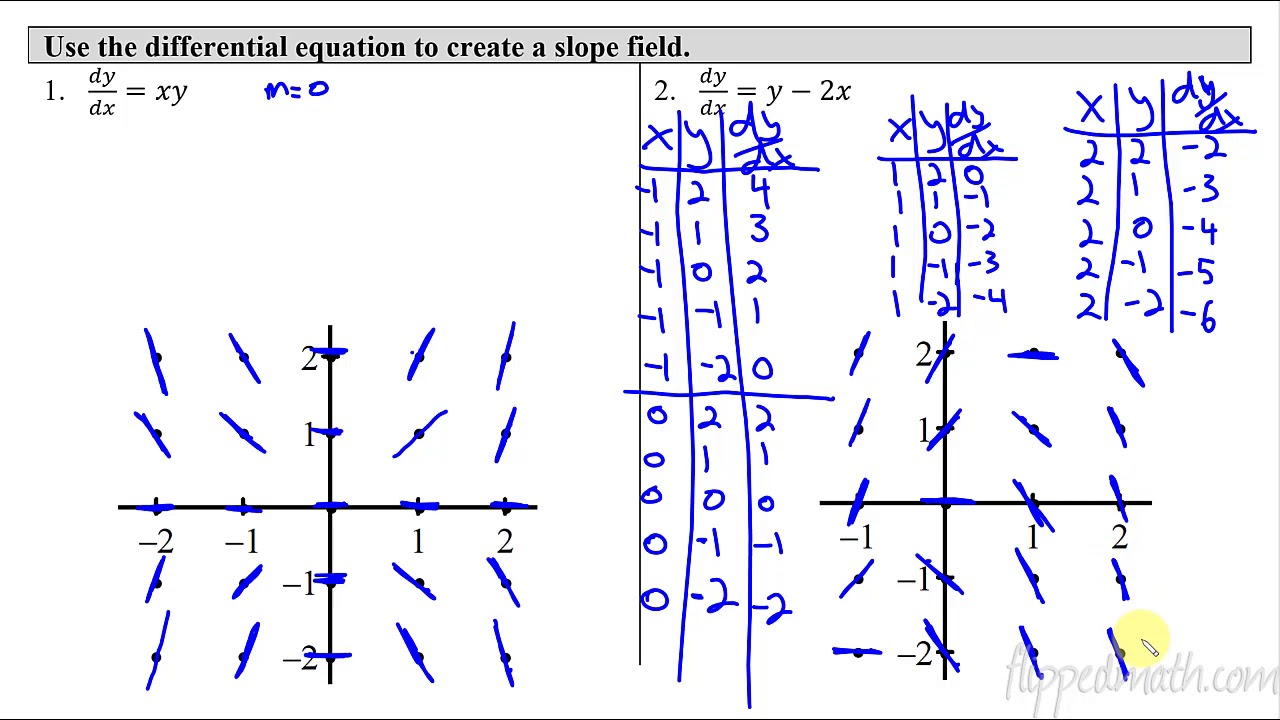
Calculus AB/BC 7.3 Sketching Slope Fields YouTube

How to sketch the slope field YouTube

Matlab How to draw slope fields by using Matlab YouTube
If You Drop A Leaf Onto This Map, Where Will It Go?
Slope Fields Are Tools Used To.
Match A Slope Field To A Solution Of A Differential Equation.
Web Let's Draw Small Pieces Of Some Of These Tangent Lines Of The Function :
Related Post: