How To Draw Vector Fields
How To Draw Vector Fields - Change the components of the vector field by typing, for example: Example 1 sketch each of the following vector fields. Web the system is autonomous (compare this section to section 1.6) and so we can draw a vector field (see end of section 3.1 ). A vector field is simply a diagram that shows the magnitude and direction of vectors (forces, velocities, etc) in different parts of space. A vector field \(\vecs{f}\) is called conservative if there exists a scalar function \(f\) such that \(\vecs \nabla f=\vecs{f}\). Let’s take a quick look at a couple of examples. Web explore math with our beautiful, free online graphing calculator. These are like functions that take in coordinates and give. The vector field can be used to represent other cases as well, that don't involve time. We will be able to visually tell what the vector field looks like and how the solutions behave, once we find the eigenvalues and eigenvectors of the matrix p. An interactive visulization of vector fields. Then, we would draw vector 〈3, 1〉 at point (4, −1). Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. A vector function is a function that takes a number of inputs, and returns a vector. Find a function f(x,y) such that f⃗ = ∇f. Web definition of vector field. We will be able to visually tell what the vector field looks like and how the solutions behave, once we find the eigenvalues and eigenvectors of the matrix p. Let’s take a quick look at a couple of examples. Web in both cases, draw a contour map of f and use gradients to draw the. Web in both cases, draw a contour map of f and use gradients to draw the vector field⃗f(x,y) = ∇f. A vector function is a function that takes a number of inputs, and returns a vector. We know about vectors, and we know about functions, so we are ready to learn about vector fields. Web let e be a set. A) is the vector fieldf⃗(x,y) = xy x2 a gradient field? →f (x,y,z) =2x→i −2y→j −2x→k f → ( x, y, z) = 2 x i → − 2 y j → − 2. A vector function is a function that takes a number of inputs, and returns a vector. A vector field is simply a diagram that shows the. Vector fields exhibit certain common shapes, which include a source (where the vectors emanate out of one point), a sink (where the vectors disappear into a hole, something. We know about vectors, and we know about functions, so we are ready to learn about vector fields. These are like functions that take in coordinates and give. For example, suppose the. Web the easiest way to make sense of the vector field model is using velocity (first derivative, output) and location, with the model of the fluid flow. →f (x,y,z) =2x→i −2y→j −2x→k f → ( x, y, z) = 2 x i → − 2 y j → − 2. To do this, draw the vector associated with a given. Web the system is autonomous (compare this section to section 1.6) and so we can draw a vector field (see end of section 3.1 ). A vector field \(\vecs{f}\) is called conservative if there exists a scalar function \(f\) such that \(\vecs \nabla f=\vecs{f}\). Change the components of the vector field by typing, for example: We can now represent a. Web definition of vector field. So to start off, let's take a very simple example, one where the vector that outputs is. The vector field f⃗(x,y) = x (x2+y2)(3/2) y (x 2+y )(3/2) # appears in electrostatics. To do this, draw the vector associated with a given point at the point in a plane. →f (x,y,z) =2x→i −2y→j −2x→k f. Web vector fields use the same amount of input dimensions as a graph, but instead of creating new dimensions for each output like a graph does, they condense the outputs into a single vector. Web the function p p, q q, r r (if it is present) are sometimes called scalar functions. Web we can sketch a vector field by. Let’s take a quick look at a couple of examples. Web let e be a set in r 3. In this case, since we divided by $z$, the magnitude of the vector field decreases as $z$ increases. F → ( x, y, z) = p ( x, y, z), q ( x, y, z), r ( x, y, z) where. For simplicity, let's keep things in 2 dimensions and call those inputs x and y. Find a function f(x,y) such that f⃗ = ∇f. We can now represent a vector field in terms of its components of functions or unit vectors, but representing it visually by sketching it is more complex because the domain of a vector field is in [latex]\mathbb{r}^2[/latex], as is the range. We know about vectors, and we know about functions, so we are ready to learn about vector fields. Vector fields and line integrals in the plane. Web let e be a set in r 3. Before we learn how to draw more vector fields, let us first show you how to find a vector associated with a given point. The vector field f⃗(x,y) = x (x2+y2)(3/2) y (x 2+y )(3/2) # appears in electrostatics. Web definition of vector field. Web this video aims to help you practise sketching vector fields in two dimensions. Web explore math with our beautiful, free online graphing calculator. F → ( x, y, z) = p ( x, y, z), q ( x, y, z), r ( x, y, z) where p, q, and r are functions of three variables. To do this, draw the vector associated with a given point at the point in a plane. Web the system is autonomous (compare this section to section 1.6) and so we can draw a vector field (see end of section 3.1 ). Example 1 sketch each of the following vector fields. For example, suppose the vector associated with point (4, −1) is 〈3, 1〉.
22+ How To Draw Vector Fields Image Ilutionis
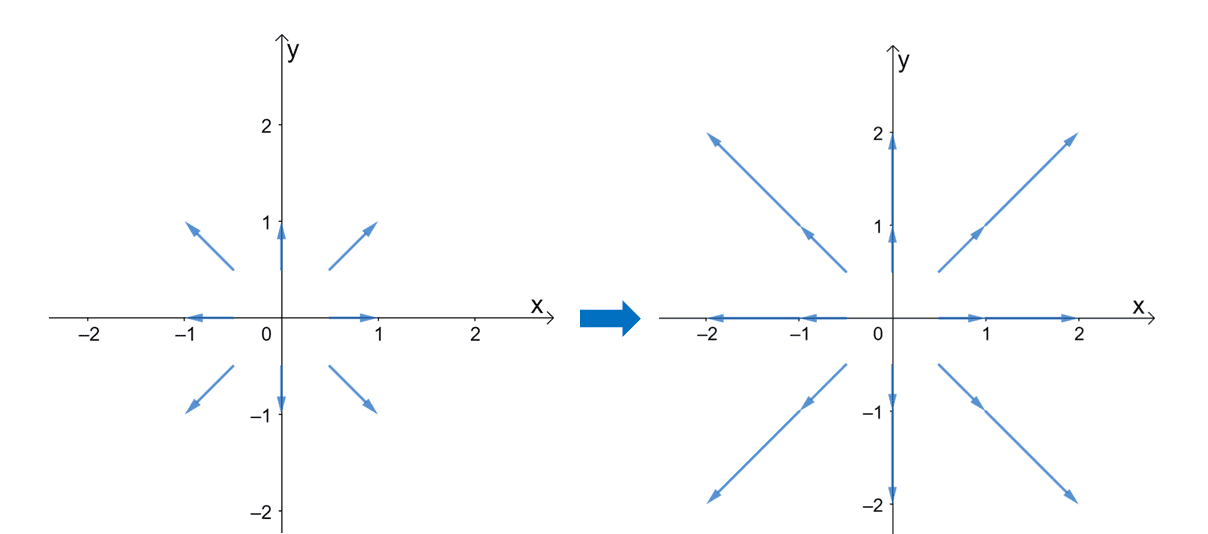
Use these vectors and sketch some of them on the xyplane to give you
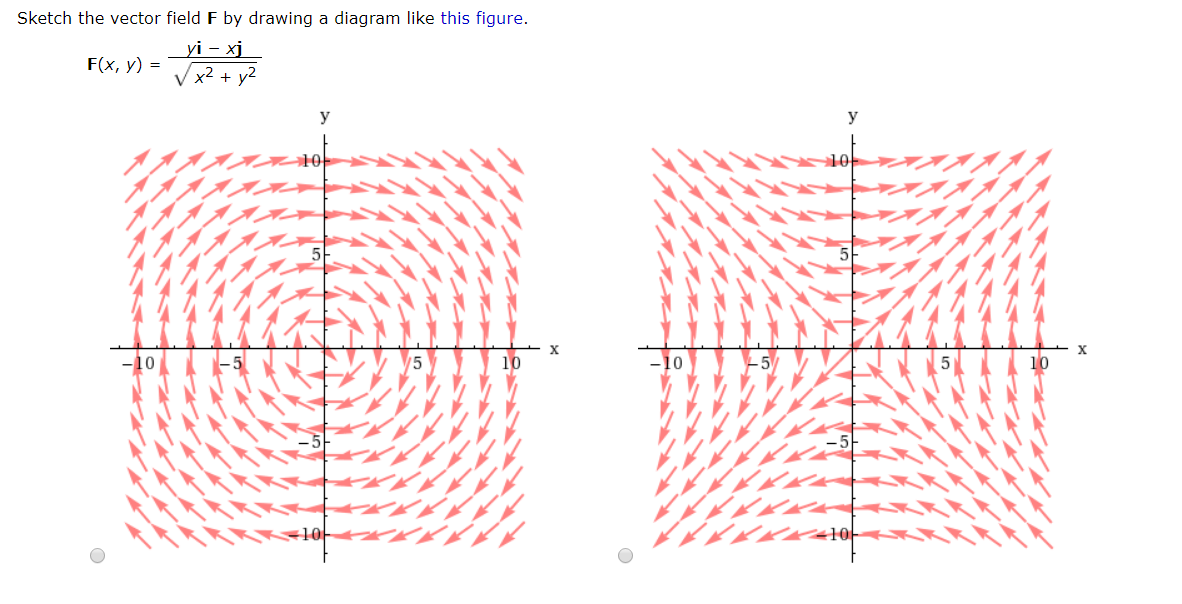
Sketch The Vector Field F By Drawing A Diagram Like This Figure Fx Y Yi

Vector Fields GeoGebra

Introduction to Vectors Definitions Components How to Draw a

HartleyMath Vector Fields
![[sketch vector fields] How to go about sketching vector fields? r](http://d2vlcm61l7u1fs.cloudfront.net/media/759/759ef035-9af6-49b5-a983-c08bf40d2c1c/phpwi9YLC.png)
[sketch vector fields] How to go about sketching vector fields? r

Example of sketching a vector field. YouTube
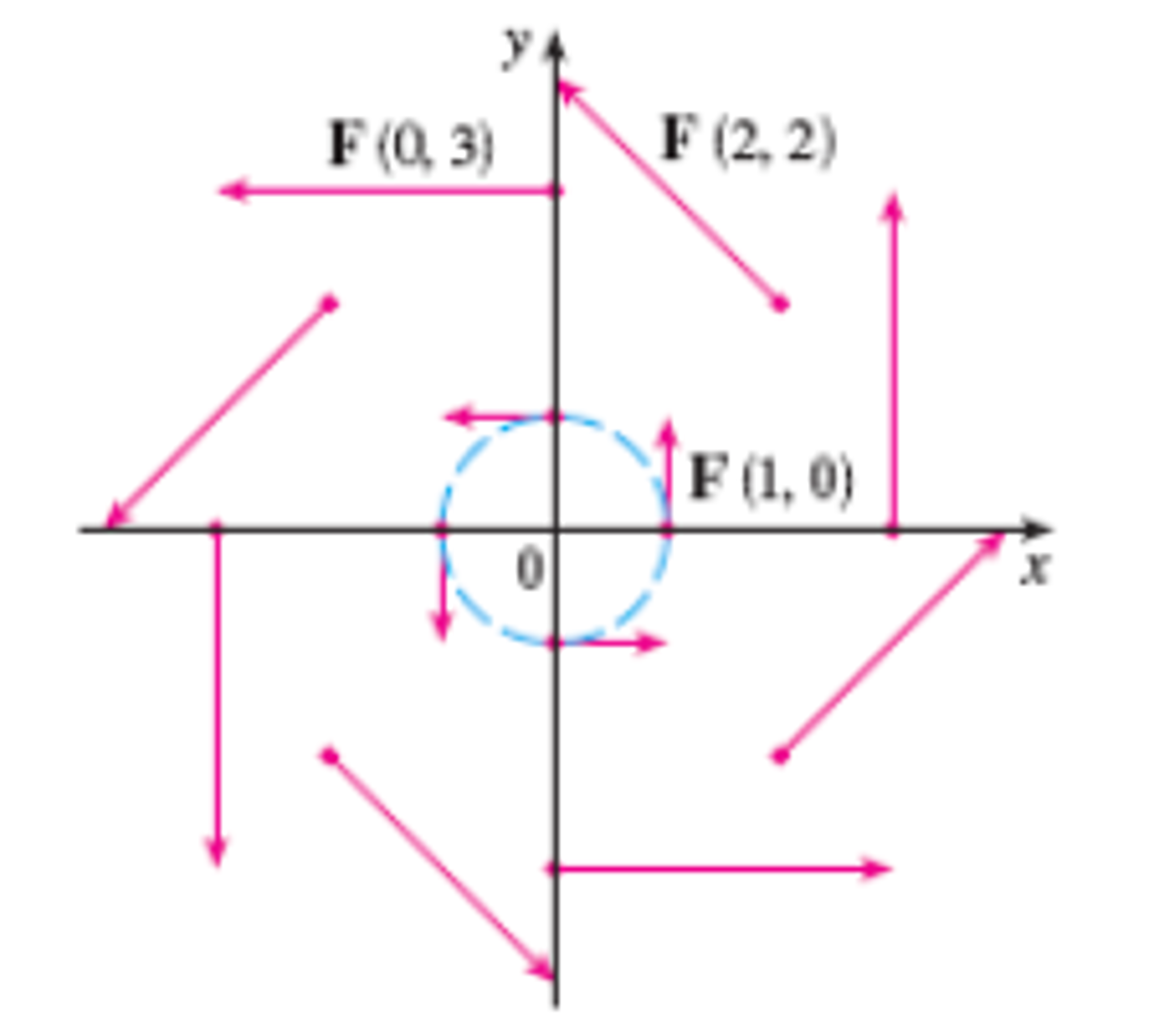
Drawing Vector Field at Explore collection of

how to draw E field vectors YouTube
→F (X,Y) =−Y→I +X→J F → ( X, Y) = − Y I → + X J →.
Web Drawing A Vector Field.
Web The Easiest Way To Make Sense Of The Vector Field Model Is Using Velocity (First Derivative, Output) And Location, With The Model Of The Fluid Flow.
Vector Fields Exhibit Certain Common Shapes, Which Include A Source (Where The Vectors Emanate Out Of One Point), A Sink (Where The Vectors Disappear Into A Hole, Something.
Related Post: