Properties Of Real Numbers Chart

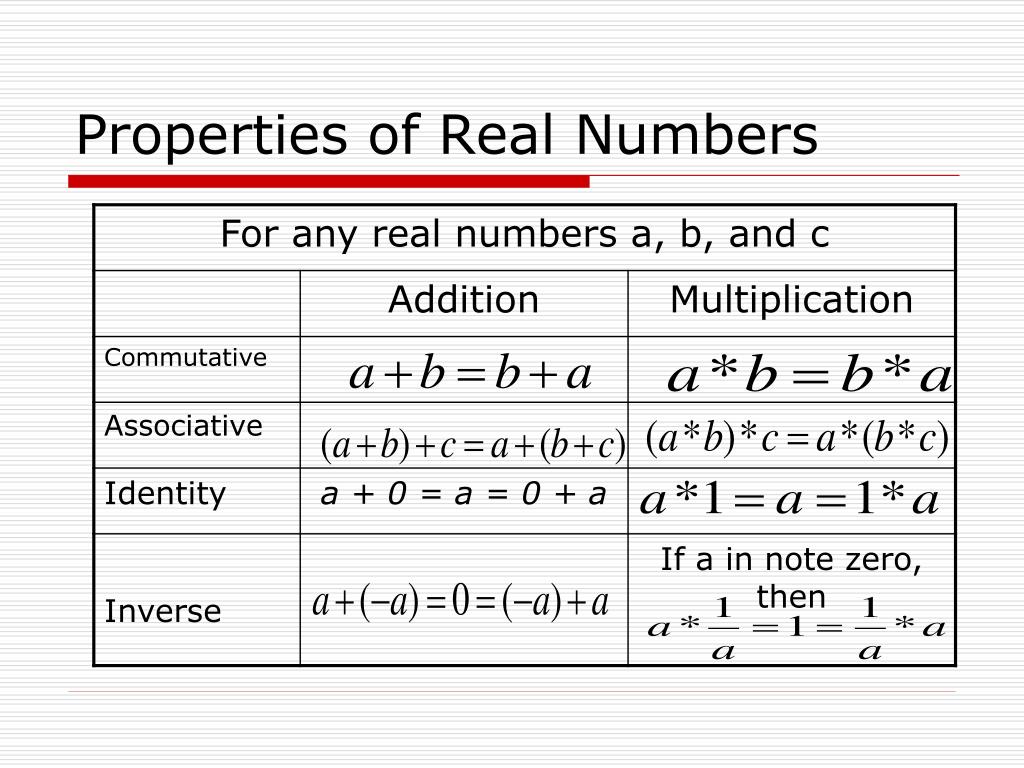
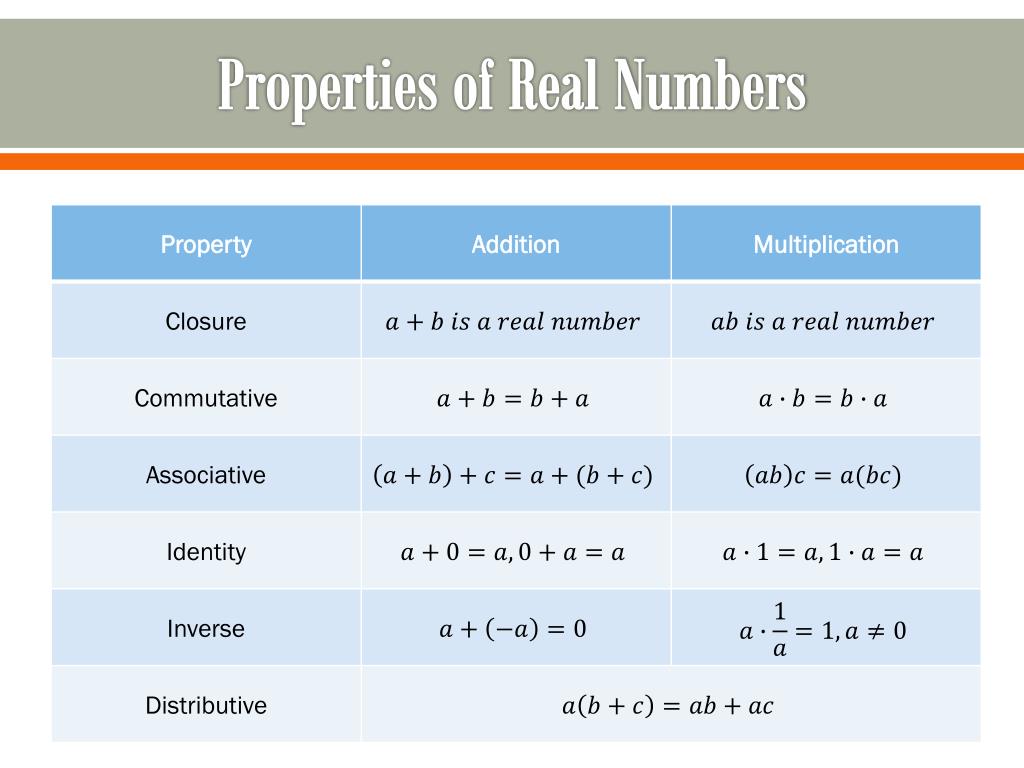
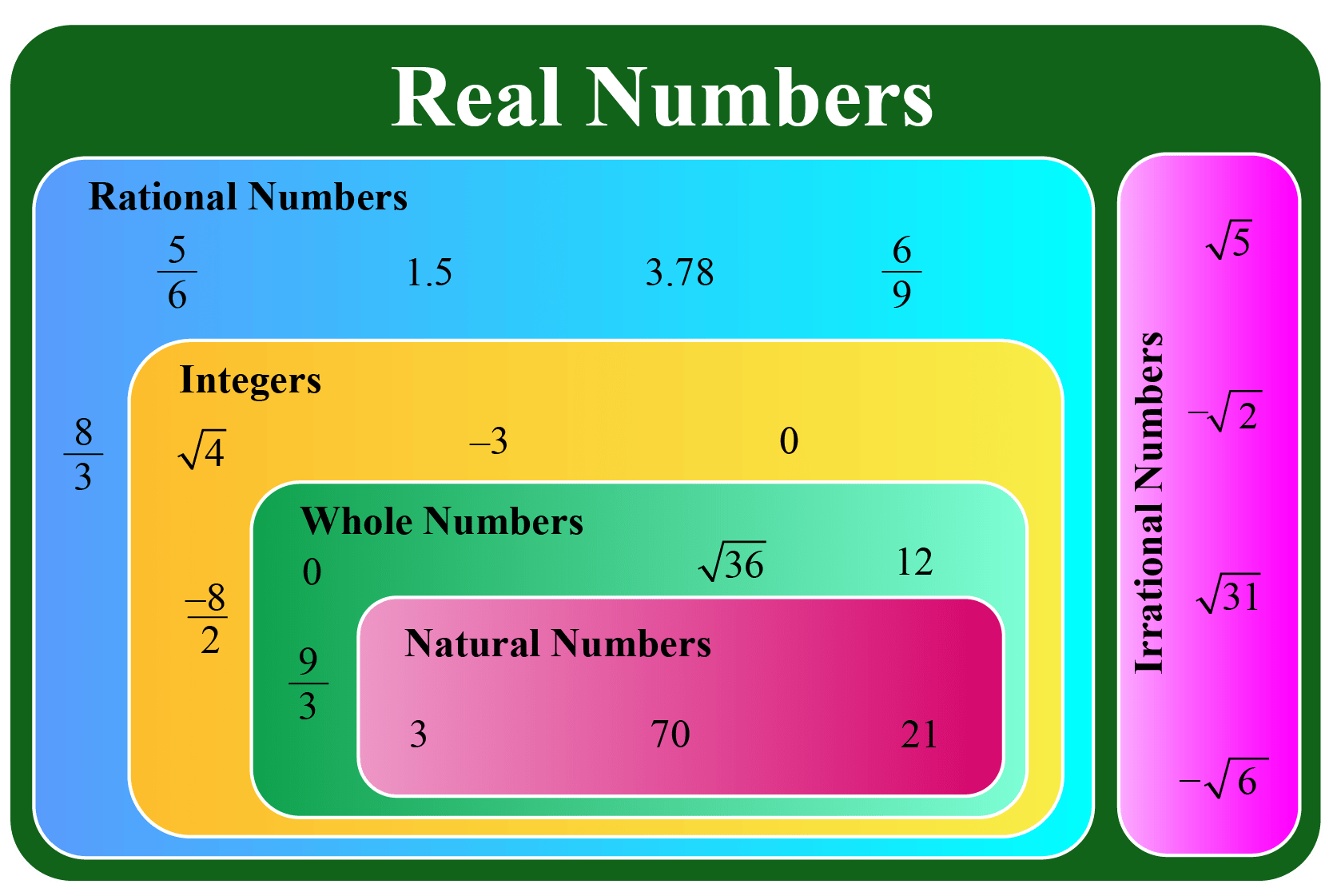
Properties Of Real Numbers Chart - Remember that when a number n is multiplied by itself, we write n2 and read it “n squared.” the result is called the square of n. Web recognizing properties of real numbers. Consider “m, n and r” are three real numbers. Web these examples illustrate the identity property of multiplication that states that for any real number \(a\), \(a\cdot 1=a\) and \(1\cdot a=a\). Addition (+), subtraction (−), multiplication (× or ·), division (÷ or /). Web real numbers include rational numbers like positive and negative integers, fractions, and irrational numbers. Additive identity property, multiplicative identity property, additive inverse property, multiplicative inverse property, commutative property of addition, commutative property of multiplication, associative property of addition, associative property of. We conclude that there is no answer to 4 ÷ 0 4 ÷ 0 and so we say that division by 0 is undefined. Web properties of real numbers. Web the properties of real numbers are introduced and applied as a culmination of the work done thus far, and to prepare students for the upcoming chapters on equations, polynomials, and graphing.

Properties Of Real Numbers

Consider “m, n and r” are three real numbers. A + b = b + a 2 + 6 = 6 + 2. There are four binary operations which take a pair of real numbers and result in another real number: Web recognizing properties of real numbers. For any real numbers and , =.

Algebra Anchor Charts Properties of Real Numbers (B&W) Math

For any real numbers and , + = +. The chart for the set of real numerals including all the types are given below: We summarize the properties of zero here. Use the commutative and associative properties. Simplify expressions with square roots.

Properties of Real Numbers Chart Pearson Connexus Support

Web these examples illustrate the identity property of multiplication that states that for any real number \(a\), \(a\cdot 1=a\) and \(1\cdot a=a\). In addition, they can be used to help explain or justify solutions. {1,2,3,.}, and also in the negative direction: Web the properties of real numbers are introduced and applied as a culmination of the work done thus far,.

PPT Properties of Real Numbers PowerPoint Presentation, free download

(a + b) + c = a + ( b + c ) (1 + 6) + 3 = 1 + (6 + 3) (ab)c = a (bc) (4 × 2) × 5 = 4 × (2 × 5) distributive example. The real numbers behave in very regular ways. Knowing these properties helps when evaluating formulas, working with equations, or.

PPT 11 Properties of Real Numbers PowerPoint Presentation, free

We conclude that there is no answer to \(4÷0\) and so we say that division by 0 is undefined. The commutative property of real numbers states that the order of numbers being added or multiplied does not change the result. Use the commutative and associative properties. Web the properties of real numbers are introduced and applied as a culmination of.

Algebra Anchor Charts Properties of Real Numbers (Color) Math

For example, 2 + 3 = 3 + 2, and 2 × 3 = 3 × 2. Additive identity property, multiplicative identity property, additive inverse property, multiplicative inverse property, commutative property of addition, commutative property of multiplication, associative property of addition, associative property of. Since any real number multiplied by 0 gives 0, there is no real number that can.

Properties of Numbers Chart TCR7742 Teacher Created Resources

Web alternatively, tap on one of the cities above to jump straight to its property values — or tap here to jump to changes to property prices in regional australia. Since any real number multiplied by 0 gives 0, there is no real number that can be multiplied by 0 to obtain 4. Web the real number system (which we.

Properties Of Real Numbers Chart

Now, which numbers are not real numbers? Web the properties of the real number system will prove useful when working with equations, functions and formulas in algebra, as they allow for the creation of equivalent expressions which will often aid in solving problems. The associative, commutative, and distributive properties of algebra are the properties most often used to simplify algebraic.

What are Real Numbers? Properties and Types of Real Numbers

Web these examples illustrate the identity property of multiplication that states that for any real number \(a\), \(a\cdot 1=a\) and \(1\cdot a=a\). Web these lessons, with videos, examples, and solutions, explain the properties of real numbers: For any real numbers and , + = +. Simplify expressions using the distributive property. Now, which numbers are not real numbers?

Algebra Anchor Charts Properties of Real Numbers (Color) Math

In addition, they can be used to help explain or justify solutions. These operations satisfy a number of rules. Web real numbers include rational numbers like positive and negative integers, fractions, and irrational numbers. Use the properties of zero. {1,2,3,.}, and also in the negative direction:

4 ∙ 5 = 5 ∙ 4.

We conclude that there is no answer to \(4÷0\) and so we say that division by 0 is undefined. Ab = ba 4 × 2 = 2 × 4. Web points to the right are positive, and points to the left are negative. For any real numbers and , =.

Being Familiar With These Properties Is Helpful In All Settings Where Numbers Are Used And.

Web what is a real number in math explained with symbol, properties, list, chart, solved examples, and diagrams. Web here are the main properties of the real numbers. The chart for the set of real numerals including all the types are given below: In addition, they can be used to help explain or justify solutions.

The Associative, Commutative, And Distributive Properties Of Algebra Are The Properties Most Often Used To Simplify Algebraic Expressions.

Web properties of real numbers chart (includes properties of equality, examples, hints) fraction properties chart (dealing with fractions) using properties of real numbers (equivalent expressions, justifying solutions) 2 + 3 = 3 + 2. Web properties of real numbers. Web in this lesson, we are going to go over the different properties of real numbers (ℜ).

Simplify Expressions Using The Distributive Property.

{1,2,3,.}, and also in the negative direction: Web these examples illustrate the identity property of multiplication that states that for any real number \(a\), \(a\cdot 1=a\) and \(1\cdot a=a\). By the end of this section, you will be able to: We summarize the identity properties below.